There’s no question that rounding to a named place or to a specific number of significant digits is fairly straightforward. However, the main question when you are looking at significant digits is to know how to round answers to the “appropriate” number of significant digits.

Let’s say that you measure a block of wood. The length is 5.6 inches, the width is 4.4 inches, and the thickness is 1.7 inches. To find the volume, you would multiply these three dimensions, to get 41.888 cubic inches. But can you actually state that you measured the volume of that block of wood to the nearest thousandth of a cubic inch? Probably not.
Check out this popular rounding calculator.
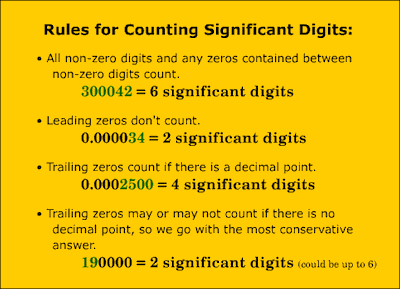
The truth is that while your measurements may be quite accurate, your tape measure was marked off in tenths of inches, and you wrote down the closest tenth of an inch that you could see. So you cannot claim five decimal places of accuracy, because none of your measurements exceeded two digits of accuracy. So, as you can easily understand, you can only give an answer with 2 significant digits in your answer. This means that instead of stating that you got 41.888 cubic inches, you needed to state that you got the result of 42 cubic inches, approximately.
Rounding Addition And Subtraction
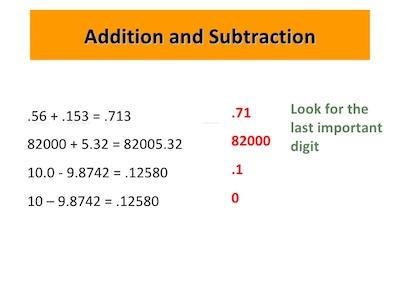
When you need to add or subtract numbers, you just need to ensure that the result of the addition or the subtraction is rounded to the same decimal place as the least-accurate number.
Here’s an example:
13.214 + 234.6 + 7.0350 + 6.38
If you look at the numbers above, you can see that the 243.6 is only accurate to the tenths place. All the other numbers are accurate to a greater number of decimal places. So:
13.214 + 234.6 + 7.0350 + 6.38 = 261.2290
Discover our round to the nearest hundredth calculator.
The digit in the tenths place is a 2, and it’s followed by another 2, so you won’t be rounding up. When you round to the tenths place, you get:
13.214 + 234.6 + 7.0350 + 6.38 = 261.2
Rounding Multiplication Or Division
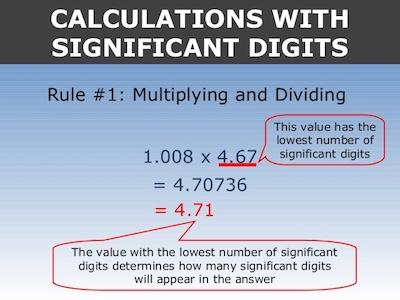
When you need to multiply or divide, you should do it as you usually do. However, as in the previous case, you will need to look at the result and round the answer to the same number of significant digits as the least-accurate number.
Use our free rounding calculator.
Here’s an example:
16.235 × 0.217 × 5
If you look at the numbers above, you can see that the number 5 has only one significant digit. So, this means that your final answer will only have one significant digit.
16.235 × 0.217 × 5 = 17.614975
Learn more about significant figures.
So, you will now need to round the result 17.614975 to one significant digit only. You will start with the 1 in the tens place; immediately to its right is a 7, which is greater than 5. So, you’ll need to round the 1 up to 2, replacing the 7 with a zero, and dropping the decimal point and everything after it. Then, you’ll get 20, which is accurate to one significant digit.
16.235 × 0.217 × 5 = 20